Eksperimentti: hyppykorkeuden määrittäminen impulssilla

Introduction

Force exerted on the force plate

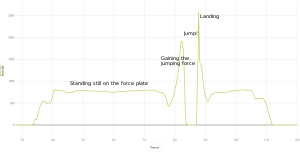
Jumping on the force plate you can feel the force. We use time of flight method to estimate the height of the jump.

Theory

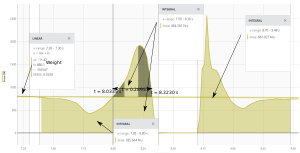
Impulse $J=\int Fdt=\Delta p=m\Delta v$. Actually $\Delta v$ is our takeoff speed because $v_{1}=0$, and we have $\Delta v=v_{0}={\frac {J}{m}}={\frac {1}{m}}\int Fdt$. Because $s=s_{0}+v_{0}t+{\tfrac {1}{2}}at^{2}$ and thus we have $h=v_{0}t-{\tfrac {1}{2}}gt^{2}$ because $h_{0}=0$ and $a=-g=-9.81m/s^{2}$. However, for the velocity we have $v=v_{0}-gt$ and at the maximum height we have that $v=0$, and thus $v_{0}=gt$ and $t={\frac {v_{0}}{g}}$. Combining these two we have

${\begin{aligned}h&=v_{0}t-{\tfrac {1}{2}}gt^{2}\\&=v_{0}{\tfrac {v_{0}}{g}}-{\tfrac {1}{2}}g\left({\frac {v_{0}}{g}}\right)^{2}\\&={\frac {v_{0}^{2}}{g}}-{\tfrac {1}{2}}{\frac {v_{0}^{2}}{g}}\\&={\frac {v_{0}^{2}}{2g}}\\&={\frac {J^{2}}{2gm^{2}}}={\frac {1}{2g}}\left({\frac {J}{m}}\right)^{2}\end{aligned}}$

Note that $J/m=v_{0}$, and thus the equation gives the correct equation.

Example

The example gives

${\begin{aligned}m&=880N/9.81=89.7kg\\J&=700Ns-89.7kg\times 9.81\times 0.2895s=700Ns-254.75Ns=445.25Ns\end{aligned}}$

and thus we have

${\begin{aligned}h&={\frac {J^{2}}{2gm^{2}}}\\&={\frac {(445.25Ns)^{2}}{2\times 9.81m/s^{2}\times (89.7kg)^{2}}}\\&={\frac {198247.5625}{315728.5716}}\\&=0.63m\\\end{aligned}}$

The takeoff velocity is $v_{0}={\frac {J}{m}}={\frac {445.25Ns}{89.7kg}}=4.96m/s$.

References

https://www.thehoopsgeek.com/the-physics-of-the-vertical-jump/

https://www.brunel.ac.uk/~spstnpl/LearningResources/VerticalJumpLab.pdf