Eksperimentti: hyppykorkeuden määrittäminen lentoajalla.: Difference between revisions
From wikiluntti
(→Theory) |
|||
| (10 intermediate revisions by the same user not shown) | |||
| Line 7: | Line 7: | ||
== Theory == | == Theory == | ||
[[File:ForcePlate jumping2 timeOfFlight.svg|thumb]] | |||
<math>s = s_0 + v_0 t + \tfrac12 at^2</math> and thus we have <math>h = v_0 t - \tfrac12 gt^2</math> because <math>h_0=0</math> and <math>a=-g = -9.81m/s^2</math>. However, for the velocity we have <math>v = v_0 - gt</math> and at the maximum height we have that <math>v=0</math>, and thus <math>v_0 = gt_m</math>. Combining these two we have | |||
<math> | <math> | ||
\begin{matrix} | |||
h &= v_0 t - \tfrac12 gt^2 \\ | |||
&= gt_m^2 - \tfrac12 gt_m^2 \\ | |||
&= \tfrac12 gt_m^2 | |||
\end{matrix} | |||
</math> | |||
The time at the maximum height (<math>t_m</math>) will be half of the flight time. | |||
== Example == | |||
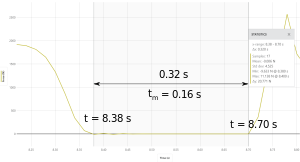
In the experiment, we have <math>t_m = 0.16</math> s, which gives <math> h = \tfrac12 gt_m^2 = \frac12 \times 9.81 \times 0.16^2 = 0.13</math> m. | |||
The takeoff velocity is <math>v_0 = gt_m = 9.81 m/s^2 \times 0.16 s = 1.5696 m/s</math>. | |||
Latest revision as of 19:12, 3 May 2022
Introduction
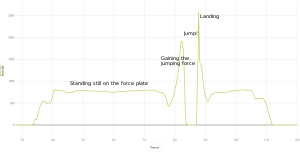
Jumping on the force plate you can feel the force. We use time of flight method to estimate the height of the jump.
Theory
and thus we have because and . However, for the velocity we have and at the maximum height we have that , and thus . Combining these two we have
The time at the maximum height () will be half of the flight time.
Example
In the experiment, we have s, which gives m.
The takeoff velocity is .











